10
INCOMMENSURABLE MAGNITUDES
HERE AGAIN IS THE THEOREM of the previous Topic:
Straight lines will have a common measure -- they will have the same ratio as natural numbers -- if and only if the squares on them have the same ratio as square numbers.
What, then, will be the case if two squares are not in the same ratio as two square numbers? What if one square is twice the size of another?
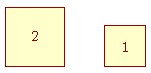
2 and 1 are not both square numbers. What must we say about the ratio of the sides?
The sides are not in the same ratio as natural numbers! One side is not a multiple of the other, any part of it, or parts of it. Those sides have no common measure. We cannot express their relationship using the normal language or arithmetic. Those sides are called incommensurable.
Problem 1.
a) If one square is three times the size of another square, are their sides
a) commensurable or incommensurable? (Commensurable means that
a) they have a common measure.)
To see the answer, pass your mouse over the colored area.
To cover the answer again, click "Refresh" ("Reload").
Incommensurable. The squares are in the ratio 3 to 1, and 3 and 1 are not both square numbers.
b) If one side is a multiple of one-eighth of an inch, could the other side
b) also be a multiple of one-eighth of an inch?
No. Those sides have no common measure.
c) If one side is a number of millionths of an inch, could the other
c) side also be a number of millionth of an inch?
No!
d) If one side is a multiple of any unit fraction, could the other side also
d) be a multiple of that unit fraction?
No.
e) Will those sides be in the same ratio as two natural numbers? That is,
e) will one side be a multiple of the other, a part of it, or parts of it?
No!
f) If one side is a rational number of units, could the other side also be a
f) rational number of those units? Explain.
No. They cannot both be rational, because if they were, they would have a common measure. (Topic 8.)
g) Can you express the ratio of those sides?
Can you?
Problem 2. How will we know when straight lines are incommensurable?
The squares on them are not in the same ratio as square numbers.
Problem 3.
a) If two squares are to one another as 9 is to 20, do their sides have a
a) common measure?
No. 9 and 20 are not both square numbers.
b) If two squares are to one another as 9 is to 25, do their sides have a
b) common measure?
Yes. What is the ratio of those sides; explicitly,
b) which multiple or part or parts of one is the other?
The smaller side is three fifths of the larger.
Problem 4. Let a square be 10 square meters.
a) Does that square have a common measure with 1 square meter?
Yes. 10 and 1 are natural numbers.
b) Will its side have a common measure with 1 meter?
No. 10 and 1 are not both square numbers.
c) Will its side be a rational number of meters?
No. The side has no common measure with 1 meter.
Problem 5.
a) If one square is four ninths of another square, is its side a fraction of
a) the other side?
Yes. 4 and 9 are square numbers.
b) If one square is four fifths of another square, is its side a fraction of
b) the other side?
No. 4 and 5 are not both square numbers.
Could both sides be a rational number of feet? No.
Problem 6. Since any two lengths could be measured, with a ruler, what sense does it make to say that two lengths are incommensurable?
Since lengths are continuous, with no units to count, we always have the problem of measuring exactly. Measurement is limited not only by the fineness of the measuring instrument, but also by the fineness of our eyes to see its readings!
| (Is it 4 | 19 64 |
or 4 | 18 64 |
?) Therefore, when we say that two lengths |
do, or do not, have a common measure, we mean as determined logically, not with rulers.
Problem 7.
a) Again, what is the ratio that natural numbers have to one another?
One number is a multiple of the other, a part of it, or parts of it; or a mixture of those.
Express the following ratios:
| 5 to 1 | 5 is five times 1. | |
| 5 to 15 | 5 is the third part of 15. | |
| 5 to 8 | 5 is five eighths of 8. | |
| 5 to 2 | 5 is two and a half times 2. | |
b) Are magnitudes necessarily in the same ratio as natural numbers?
No! We cannot always express their relationship in words.
c) Therefore, what do we mean by the "ratio" of two magnitudes?
? ? ?
The new theory of proportions
Incommensurability completely upsets the theory of proportions. For if
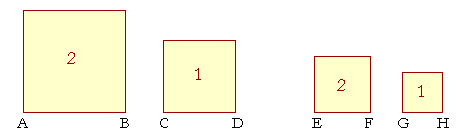
the square on AB is twice the square on CD, if they are in the ratio 2 : 1, then the lengths AB, CD are incommensurable; 2 is not a square number. And if the square on EF is also twice the square on GH, then EF, GH are also incommensurable -- yet we expect that whatever relationship AB has to CD, EF will have it to GH. We expect, proportionally,
AB : CD = EF : GH.
That is,
AB is to CD in the same ratio as EF is to GH.
But according to the definition of natural numbers being "in the same ratio," that will make no sense, because AB is not any multiple of CD, any part of it or any parts of it!
Yet we can see that they have the same relationship. Therefore we must create a new definition of "in the same ratio," one that will be applicable to incommensurable magnitudes. We will not present the new definition here. Seeing the need for it -- namely, the discovery of incommensurables -- is the climax of our present study. Historically, the new definition of "in the same ratio" has always marked the beginning of advanced mathematics.
Next Topic: Irrational numbers
www.proyectosalonhogar.com
