2
PROPORTIONS
This Lesson continues from Lesson 1.
The theorem of the alternate proportion
The theorem of the same multiple
The theorem of the common divisor
Proportions
A proportion is a statement that two ratios are the same.
5 is to 15 as 8 is to 24.
5 is the third part of 15, just as 8 is the third part of 24.
We will now introduce this symbol 5 : 15 to signify the ratio of 5 to 15. A proportion will then appear as follows:
5 : 15 = 8 : 24.
"5 is to 15 as 8 is to 24."
Problem 1. Read the following. Why is each one a proportion?
a) 2 : 6 = 10 : 30
"2 is to 6 as 10 is to 30." Because 2 is the third part of 6, just as 10 is the third part of 30.
b) 12 : 3 = 24 : 6
"12 is to 3 as 24 is to 6." Because 12 is four times 3, just as 24 is four times 6.
c) 2 : 3 = 10 : 15
"2 is to 3 as 10 is to 15." Because 2 is two thirds of 3, just as 10 is two thirds of 15.
Problem 2. Complete each proportion.
| a) 1 : 2 = 5 : 10 | b) 3 : 9 = 10 : 30 | c) 4 : 1 = 20 : 5 | ||
| d) 49 : 7 = 56 : 8 | e) 6 : 42 = 9 : 63 | f) 18 : 2 = 45 : 5 | ||
| g) 2 : 3 = 10 : 15 | h) 3 : 4 = 75 : 100 | i) 2 : 5 = 20 : 50 | ||
| Problem 3. |  |
AB, CD are straight lines, and AB is three fifths of CD. Express that relationship as a proportion.
AB : CD = 3 : 5
Example 1. If, proportionally, a : b = 3 : 4, then, explicitly, what ratio has a to b?
Answer. The proportion implies the ratio of a to b, but it does not state that ratio explicitly. What ratio has 3 to 4? 3 is three fourths of 4. Explicitly, then, that is the ratio of a to b. a is three fourths of b.
Proportions imply ratios.
Problem 4. Explicitly, what ratio has x to y?
a) x : y = 1 : 5. x is the fifth part of y.
b) x : y = 32 : 8. x is four times y.
c) x : y = 7 : 10. x is seven tenths of y.
The theorem of the alternate proportion
The numbers in a proportion are called the terms: the 1st, the 2nd, the 3rd, and the 4th.
1st : 2nd = 3rd : 4th
We say that the 1st and the 3rd are corresponding terms, as are the 2nd and the 4th.
The following is the theorem of the alternate proportion:
|
If four numbers are proportional, then the corresponding terms are also proportional. That is, as the first term is to the third, so the second will be to the fourth. |
| If |
| a : b = m : n, |
| then, alternately, |
| a : m = b : n. |
(Euclid, VII. 13.)
For example, since
1 : 3 = 5 : 15,
then alternately,
1 : 5 = 3 : 15.
(Skill in Arithmetic: Lesson 17, Question 2.)
Problem 5. State the alternate proportion.
| a) | 10 : 30 = 5 : 15 | b) | 2 : 8 = 6 : 24 | |
| 10 is to 5 as 30 is to 15. | 2 is to 6 as 8 is to 24. | |||
| c) | 2 : 3 = 10 : 15 |
| 2 is to 10 as 3 is to 15. | |
This leads to:
The theorem of the same multiple
Let us complete this proportion,
4 : 5 = 12 : ?
4 is four fifths of 5 (Lesson 1), but it is not obvious of what number 12 is four fifths.
Alternately, however, 4 is the third part of 12 -- or we could say that 4 has been multiplied by 3. Therefore, 5 also must be multiplied by 3 --
4 : 5 = 12 : 15
That is,
4 : 5 = 3 × 4 : 3 × 5.
This is called the theorem of the same multiple.
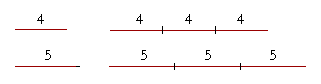
4 is four fifths of 5. But each 4 has that same ratio to each 5. Two 4's, then, upon adding them, will have that same ratio to two 5's:
Four fifths of 5 + Four fifths of 5 = Four fifths of (5 + 5).
(Lesson 15 of Arithmetic.) Three 4's will have that same ratio to three 5's. And so on. Any number of 4's will have that same ratio, four fifths, to an equal number of 5's.
|
If two numbers are multiplied by the same number, then the products will have the same ratio as the numbers multiplied. |
(Euclid, VII. 17.)
Problem 6. Write five pairs of numbers that have the same ratio as 3 : 4.
Generate them by taking the same multiple of both 3 and 4. For example,
6 : 8, 9 : 12, 12 : 16, 15 : 20, 18 : 24
Problem 7. Complete each proportion.
| a) 4 : 9 = 8 : 18 | b) 4 : 9 = 12 : 27 | c) 4 : 9 = 16 : 36 | ||
| d) 7 : 8 = 21 : 24 | e) 9 : 5 = 63 : 35 | f) 4 : 11 = 20 : 55 | ||
| g) 2 : 9 = 16 : 72 | h) 6 : 5 = 54 : 45 | i) 8 : 3 = 56 : 21 | ||
Problem 9. Complete this proportion, 2.45 : 7 = 245 : 700.
Since 2.45 has been multiplied by 100, then 7 also must be multiplied by 100.
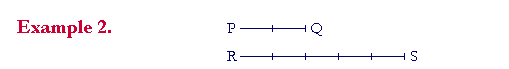
PQ is two fifths of RS. If PQ is 12 miles, then how long is RS?
Solution. Since PQ is two fifths of RS, then proportionally,
PQ : RS = 2 : 5.
If PQ is 12 miles, then
PQ : RS = 2 : 5 = 12 miles : ? miles.
That is, 12 miles corresponds to PQ. And since 12 is 6 × 2, the missing term is 6 × 5:
PQ : RS = 2 : 5 = 12 miles : 30 miles.
RS is 30 miles.
Or, since
PQ : RS = 2 : 5,
then inversely,
RS : PQ = 5 : 2.
Now, what ratio has 5 to 2? 5 is two and a half times 2. RS therefore is two and a half times PQ. And if PQ is 12 miles, then RS is 24 + 6 = 30 miles.
| Problem 10. |  |
AB, CD are straight lines, and AB is three fourths of CD. Specifically, AB is 24 cm. How long is CD?
AB : CD = 3 : 4 = 24 cm : ? Since 24 is 8 × 3, the missing term is 8 × 4 = 32 cm.
The theorem of the common divisor
Since we may multiply both terms by the same number, then, symmetrically, we may divide both terms by the same number.
25 : 40 = 5 : 8
upon dividing both 25 and 40 by 5.
Explicitly, then, we see that 25 is five eighths of 40.
Problem 11. Explicitly, what ratio has 16 to 40? Express that ratio so that the terms have no common divisors (except 1).
Upon dividing both terms by 8, 16 : 40 = 2 : 5.
Explicitly, 16 is two fifths of 40.
When the terms of a ratio have no common divisors except 1, then we have expressed that ratio with the lowest terms. They are the smallest terms that have that ratio.
Problem 12. Explicitly, what ratio have the following? Express each ratio with the lowest terms.
a) 6 is three fourths of 8, upon dividing each term by 2.
| b) 12 is two thirds of 18. | c) 10 is two fifths of 25. | |
| d) 9 is three eighths of 24. | e) 6 is three fifths of 10. | |
| f) 90 is nine tenths of 100. | g) 72 is eight ninths of 81. | |
| h) 16 is four fifths of 20. | i) 21 is three tenths of 70. | |
The language of ratio
Example 1. Joan earns $1600 a month, and pays $400 for rent. Express that fact in the language of ratio.
Answer. "A quarter of Joan's salary goes for rent."
That sentence, or one like it, expresses the ratio of $400 to $1600, of the part that goes for rent to her whole income. We are not concerned with the numbers themselves, but only their ratio.
Example 2. In Erik's class there are 30 pupils, while in Ana's there are only 10. Express that fact in the language of ratio.
Answer. "In Erik's class there are three times as many pupils as in Ana's."
This expresses the ratio of 30 pupils to 10.
Example 3. In a class of 24 students there were 16 B's. Express that fact in the language of ratio.
Answer. "Two thirds of the class got B."
This expresses the ratio of the part that got B to the whole number of students; 16 out of 24. Their common divisor is 8. 8 goes into 16 two times and into 24 three times. 16 is two thirds of 24.
Problem 13. Express each of the following in the language of ratio. Use a complete sentence.
a) In a class of 30 pupils, there were 10 A's.
A third of the class got A.
b) Out of 120 people surveyed, 20 responded No.
A sixth of the people surveyed responded No.
c) The population of Eastville is 60,000, while the population of
d) Westville is 20,000.
The population of Eastville is three times the population of Westville.
d) Over the summer, John saved $1000, while Bob has saved only $100.
Over the summer, John saved ten times more than Bob.
e) At a party, there were 12 girls and 4 boys.
At that party, there were three times as many girls as boys.
f) In a class of 28 students, there were 21 A's.
Three fourths of the students got A.
g) In a survey of 60 people, 40 answered Yes.
Two thirds of the people surveyed answered Yes.
h) In a class of 40 pupils, 25 got a B.
Five eighths of the pupils got B.
i) Of the 2100 students who voted, 1400 voted for Harrison.
Two thirds of the students voted for Harrison.
j) This month's bill is $50, while last month's was only $20.
This month's bill is two and a half times last month's.
k) Sabina makes $24,000 a year, while Clara makes only $16,000.
Sabina makes one and a half times what Clara makes.
l) In the past thirty years, the population grew from 20,000 to 70,000.
In the past thirty years, the population grew three and a half times.
Next Topic: Continuous versus discrete
www.proyectosalonhogar.com
