34
THE SLOPE OF A
STRAIGHT LINE
Parallel and perpendicular lines
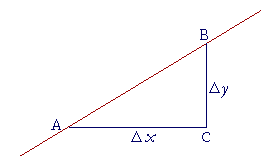
ON THAT STRAIGHT LINE, let us move from the point A to the point B. But we will not move along the line -- we will move over to C and then up to B, where ACB is a right angle.
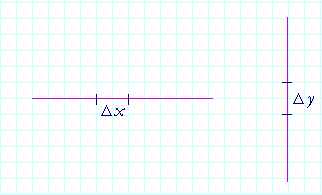
By the symbol Δx ("delta x") we mean the horizontal leg of that triangle, and by the symbol Δy ("delta y") we mean the vertical leg. Then by the slope of that line we mean this number:
| Slope | = | _Vertical leg_ Horizontal leg |
= | Δy Δx |
This number is often call the "rise over the run."
For example:
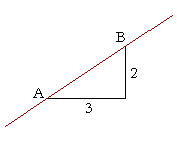
| If we move over 3 and up 2, then the slope of that line is | 2 3 |
. |
That means that for every 3 units the line moves over, it moves up 2. This will be true between any two points on the line -- over 6 and up 4 -- because a straight line has only one slope. (Topic 8 of Precalculus.)
Up or down?

Which line is sloping "up"? And which line is sloping "down"?
To move from the point A to point B, we move over 2 and up 3.
| The slope of that line is | 3 2 |
. But to move from C to D, we must go over 2 |
| and down 3. We say that the slope of that line is − | 3 2 |
. |
The line on the left is sloping "up," and its slope will be a positive number. The line on the right is sloping "down." Its slope will be a negative number.
For, we should always move from left to right, so that Δx is always positive. When the slope is positive, Δy will be positive. But when the slope is negative, Δy will be negative.
Problem 1. What number is the slope of each line?
To see the answer, pass your mouse over the colored area.
To cover the answer again, click "Refresh" ("Reload").
Do the problem yourself first!
| a) |  |
b) | 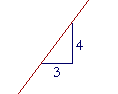 |
|||||
| 3 4 |
4 3 |
|||||||
| c) | 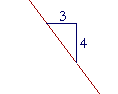 |
d) | 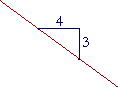 |
|||||||
| − | 4 3 |
− | 3 4 |
|||||||
Horizontal and vertical lines
What is the slope of a horizontal line -- that is, a line parallel to the x-axis? And what is the slope of a vertical line?
A horizontal line has slope 0, because as we move along the line, the line does not move up or down -- Δy = 0.
| Δy Δx |
= | 0 Δx |
= | 0 | (Lesson 6) |
For a vertical line, however, the slope is not defined. The slope tells how the y-coordinate changes when the x-coordinate changes. But the x-coordinate does not change -- Δx = 0. A vertical line does not have a slope.
| Δy Δx |
= | Δy 0 |
= | No value. | (Lesson 6) |
Problem 2.
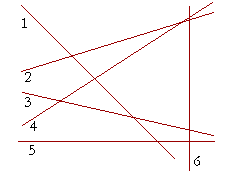
a) Which numbered lines have a positive slope? 2 and 4.
b) Which numbered lines have a negative slope? 1 and 3.
c) What slope has the horizontal line 5? 0.
c) What slope has the vertical line 6? It does not have a slope.
Example 1. Calculate the slope of the line that passes through the points (3, 6) and (1, 2)
Solution. To do this problem, let us state the definition of the slope as follows. It is the number
| Δy Δx |
= | Difference of y-coordinates Difference of x-coordinates |
= |
Therefore, the slope of the line passing through (3, 6) and (1, 2) is:
| Δy Δx |
= | 6 − 2 3 − 1 |
= | 4 2 |
= 2 |
Note: It does not matter which point we call the first and which the second. But if we calculate Δy starting with (3, 6), then we must calculate Δx also starting with (3, 6).
Problem 3. Calculate the slope of the line that joins these points.
| a) | (1, 5) and (4, 17) | b) | (−3, 10) and (−2, 7) | |||||||||
| 17 − 5 4 − 1 |
= | 12 3 |
= | 4 | _ 10 − 7 _ −3 − (−2) |
= | 3 −1 |
= | −3 | |||
| c) | (1, −1) and (−7, −5) | d) | (2, −9) and (−2, −5) | |||||||||
| −5 − (−1) −7 − 1 |
= | −4 −8 |
= | ½ | −9 − (−5) 2 − (−2) |
= | −4 4 |
= | −1 | |||
The slope-intercept form
This linear form
y = ax + b
is called the slope-intercept form of the equation of a straight line. Because, as we can prove (Topic 9 of Precalculus): a is the slope of the line, and b is the y-intercept.
Problem 4. What number is the slope of each line, and what is the meaning of each slope?
a) y = 2x + 1
The slope is 2. This means that for every 1 unit x increases, y increases 2 units. Over 1, and up 2.
| b) y = − | 2 3 |
x + 4 |
| The slope is − | 2 3 |
. This means that for every 3 units x |
increases, y decreases 2 units. Over 3 and down 2.
Problem 5.
a) Write the equation of the straight line whose slope is 3 and whose
a) y-intercept is 1.
y = 3x + 1
b) Write the equation of the straight line whose slope is −1 and whose
a) y-intercept is −2.
y = −x − 2
| c) | Write the equation of the straight line whose slope is | 2 3 |
and which |
| passes through the origin. | |||
| y = | 2 3 |
x. The y-intercept b is 0. |
Problem 6. Sketch the graph of y = −2x.
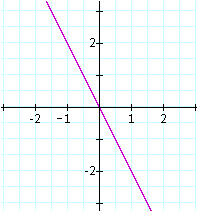
This is a straight line of slope −2 -- over 1 and down 2 -- that passes through the origin: b = 0.
(Compare Lesson 33, Problem 15.)
The general form
This linear form
Ax + By + C = 0
where A, B, C are integers (Lesson 2), is called the general form of the equation of a straight line.
Problem 7. What number is the slope of each line, and what is the meaning of each slope?
a) x + y − 5 = 0
This line is in the general form. It is only when the line is in the slope-intercept form -- y = ax + b -- that the slope is a. Therefore, on solving for y: y = −x + 5. The slope therefore is −1. This means that for every 1 unit the line goes over, it goes down 1.
| b) | 2x − 3y + 6 | = | 0 | |||
| −3y | = | −2x − 6 | ||||
| y | = | 2 3 |
x + 2, | on dividing every term by −3. | ||
| The slope therefore is | 2 3 |
. This means that for every 3 units |
the line goes over, it goes up 2.
| c) | Ax + By + C | = | 0 | |||||
| By | = | −Ax − C | ||||||
| y | = | − | A B |
x | − | C B |
, on dividing every term by B. | |
| The slope is − | A B |
. |
We can view this as a formula for the slope when the equation is in the general form. Thus, if the equation is
4x − 5y + 2 = 0,
then the slope is
| − | 4 −5 |
= | 4 5 |
. |
Parallel and perpendicular lines
Straight lines will be parallel if they have the same slope. Thus the following are parallel lines:
y = 3x + 1 and y = 3x − 8.
They have the same slope 3.
Straight lines will be perpendicular if 1) they have opposite signs -- one positive and one negative, and 2) they are reciprocals on one another.
That is:
| If m is the slope of one line, then a perpendicular line has slope − | 1 m |
. |
To be specific, if a line has slope 4, then every line that is perpendicular to it has slope −¼.
(We will prove that below.)
Problem 8. Which of these lines are parallel and which are perpendicular?
| a) y = 2x + 3 | b) y = −2x + 3 | c) y = ½x + 3 | d) y = 2x − 3 |
a) and d) are parallel. b) and c) are perpendicular.
Problem 9. If a line has slope 5, then what is the slope of a
| perpendicular line? | − |
1 5 |
| Problem 10. If a line has slope − | 2 3 |
, then what is the slope of a | |
| perpendicular line? |
3 2 |
||
| Problem 11. If a line has equation y = 6x − 5, then what is the slope | ||
| of a perpendicular line? | − |
1 6 |
The slopes of perpendicular lines
If two straight lines are perpendicular to one another, then the product of their slopes is −1.
That is: If the slope of one line is m, then the slope of the perpendicular line
| is − | 1 m |
. |
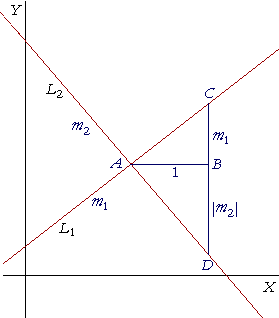
Let L1 be a straight line, and let the perpendicular straight line L2 cross L1 at the point A.
Let L1 have slope m1, and let L2 have slope m2. Assume that m1 is positive. Then m2, as we will see, must be negative.
Since a straight line has one slope (Theorem 8.1 of Precalculus),
we may draw AB parallel to the x-axis equal to 1, and BC at right angles to AB equal to m1.
Extend CB in a straight line to join L2 at D.
Then the length of BD will be |m2|, for in going from A to D, we go over 1 and down |m2|.
That is, m2 is a negative number.
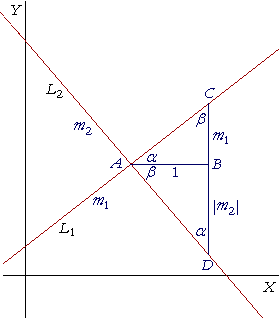
(Same figure.)
Now, since angle CAD is a right angle, the angle a is the complement of angle b.
But triangle ABD is right-angled, and therefore the angle at D is also the complement of angle b;
therefore the angle at D is equal to a.
The right triangles ABC, ABD therefore are similar (Topic 5 of Trigonometry),
and the sides opposite the equal angles are proportional:
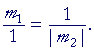
This implies
m1 |m2| = 1.
But m2 is negative. Therefore,
m1m2 = −1.
That is what we wanted to prove.
Next Lesson: Simultaneous linear equations
www.proyectosalonhogar.com
